Rigid Body
Along with static meshes, objects with rigid bodies are most commonly used. Assigning an objects rigid body together with collision shape ensures that it moves and realistically interacts with other objects according to rigid bodies dynamics. Representing objects as rigid bodies is the most effective in terms of performance.
It is characterized by total mass of all its shapes, damping of linear and angular velocities etc. In addition to that, it is possible to control the undermentioned parameters.
See also
Shape-based mass parameters
The rigid body is comprised of shapes that approximate its volume. A shape has a mass (and density), a center of mass and inertia tensor calculated on the base of the previous two. As for the whole body, these parameters of each shape and shape positioning within the body can be used to compute the body's total mass and inertia properties. This computation is automatically done if Shape-based mass parameters box is checked.
Setting the individual masses or densities of the shapes is the most intuitive method to achieve correct physical behavior of the body. Even if overridden by body parameters, they are still used to determine the distribution of mass within the object.
Overriding Shapes Parameters
Unchecking the Shape-based mass parameters box allows to redefine some total parameters for the body: corresponding fields are activated where the custom values are set up manually. For example, body's mass overrides the calculated sum of all the shapes masses.
Center of mass
An arbitrary point can be set to be a center of mass of the body. It may be necessary to correct the motion for more desirable and pleasing to the eye. For example, tilting doll with a sphere shape requires an uneven mass distribution: its weight should be centered closer to the bottom, so it always stands up when tilted.
Center of mass is set as coordinates of the point in local space of the body.
Inertia
The moment of inertia describes the resistance to rotation of the body in different directions. It is a rotational analog of mass for linear motion. However, it's not only related to the total mass of the body, but also to the distribution of the mass throughout the body. The farther from the axis of rotation is, the more rotational inertia the body has. For example, imagine rotating a dumbbell around the center: if the weights are far on the opposite sides, the speed of rotation decreases. And vice versa, bringing the weights closer to the center results in faster and more effortless spinning.
The inertia tensor that allows to compute a moment of inertia about arbitrary axis is represented as a matrix, I:
| Ixx | Ixy | Ixz |
| Iyx | Iyy | Iyz |
| Izx | Izy | Izz |
Here Ixx sets the moment of inertia around theX-axis when the body is rotated around the X-axis, Ixy sets the moment of inertia around theY-axis when the objects are rotated around the X-axis, etc. As for values:
- The higher the moment of inertia value, the more torque should be be applied to rotate the body about a particular axis. Conversely, the lower the value, the easier it is to change the angular velocity of a body about a particular axis. Values are corrected with respect to the average value to provide smoother movement.
- To rotate the body evenly about the rotation axis, the matrix should be diagonal, i.e all values outside the main diagonal are set to zero. Otherwise, the body may start to rotate chaotically.
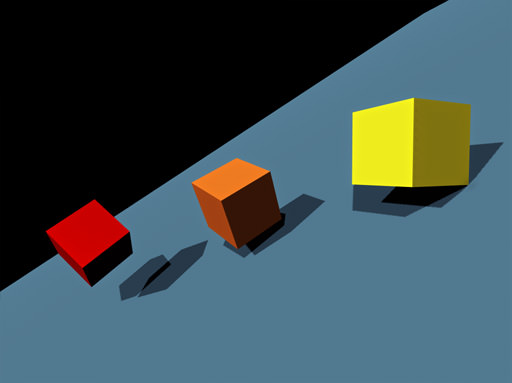
For example, the following illustration shows three boxes with different inertia tensors. The yellow box has very high inertia:
| 100 | 0 | 0 |
| 0 | 100 | 0 |
| 0 | 0 | 100 |
Because of that, it can only slide down when falling upon the tilted plane. The red box, on the contrary, has the lowest inertia and rotates most eagerly.
Freezable Body
If a Freezable flag is set for a rigid body, its simulation is freezed when necessary.